As we look out into the universe we see structure on a wide range of physical scales. The most obvious example is that stars cluster in groups and galaxies. Early redshift surveys of the 1980s showed that these galaxies are also not distributed randomly throughout the Universe. They are found to lie in clusters, filaments, bubbles and sheet like structures. There exist large regions of the Universe which contain almost no galaxies. The nearby universe then shows a large amount of structure on the very largest scales.
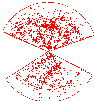The positions of galaxies in the Center for Astrophysics (CfA) redshift
survey. The plot shows a thin slice through the universe (the pie shapes)
containing nearly 10,000 galaxies.
The observer is situated at the narrow end of the slice. Distances radially
outward indicate the observed redshift of a galaxy.
Recent very large scale galaxy surveys have now reached far enough out into our local volume to begin to see the end of greatness. That is they sample a large enough volume of space that the largest structures in the survey (about 100Mpc in size) are no longer of the same size of the survey itself.
Galaxy surveys with well defined selection criteria enable us to extract information about the clustering pattern. Such surveys are driven in part by the map-makers instinct: the desire to discover and name the structures which we can see through our telescopes. They are also driven by a more theoretical desire: they provide the data with which we can test our ideas about the origin of structure in the universe.
To characterize the distribution of galaxies a number of statistical tools have been developed. The most widely used approach to quantifying the degree of clustering observed is to measure the correlation functions. For example the two-point correlation function is the probability, in excess of random, of finding a galaxy at a fixed distance from a random neighbor. Its Fourier transform is the power spectrum (which is now more widely used). Beyond this one investigates higher order correlation functions, for example the distribution of counts-in-cells: the distribution of the number of galaxies found in cells of a given size which one lays down atop the survey.
