It is most useful to describe the CMB anisotropy on the celestial sphere by spherical-harmonic multipole moments,
![]()
The multipole moments, which are determined by the underlying
density perturbations, can only be described statistically.
They have zero mean,
i.e., ![]() , and if the underlying density fluctuations
are described by a gaussian random process, as inflation
predicts, the angular power spectrum,
, and if the underlying density fluctuations
are described by a gaussian random process, as inflation
predicts, the angular power spectrum,
![]() ,
contains all possible information.
(The angled brackets indicate the average over all observers
in the Universe; the absence of a preferred direction in the Universe
implies that
,
contains all possible information.
(The angled brackets indicate the average over all observers
in the Universe; the absence of a preferred direction in the Universe
implies that ![]() is independent of m.)
If the density fluctuations are nongaussian, as other models predict,
then higher-order correlations functions contain additional information.
is independent of m.)
If the density fluctuations are nongaussian, as other models predict,
then higher-order correlations functions contain additional information.
Temperature differences between points on the sky separated
by angle ![]() are related to those multipoles with spherical-harmonic
indices around
are related to those multipoles with spherical-harmonic
indices around ![]() . The rms fractional
temperature fluctuation for a given angular separation is then
. The rms fractional
temperature fluctuation for a given angular separation is then
![]()
The angle ![]() subtends a length on the surface of last scattering
That would now, by the Hubble expansion of the universe, be about
200Mpc per degree (1Mpc is approximately 3 million light-years).
Therefore, the corresponding
subtends a length on the surface of last scattering
That would now, by the Hubble expansion of the universe, be about
200Mpc per degree (1Mpc is approximately 3 million light-years).
Therefore, the corresponding ![]() th multipole is determined by density
fluctuations on that wavelength scale.
For example, the density fluctuations of
wavelength around 2Mpc, which seed galaxies, subtend an angle
th multipole is determined by density
fluctuations on that wavelength scale.
For example, the density fluctuations of
wavelength around 2Mpc, which seed galaxies, subtend an angle ![]() of around an arcminute; those of 20Mpc that seed clusters of galaxies
subtend about 10 arcminutes; and those of around 200Mpc
that seed the largest structures seen today subtend about 1 degree.
(All of these distances were a thousand times smaller at the time of
last scattering, when the linear size of the universe was a thousand times
smaller. But it is conventional to quote ``comoving separations'' as they
would be now.)
of around an arcminute; those of 20Mpc that seed clusters of galaxies
subtend about 10 arcminutes; and those of around 200Mpc
that seed the largest structures seen today subtend about 1 degree.
(All of these distances were a thousand times smaller at the time of
last scattering, when the linear size of the universe was a thousand times
smaller. But it is conventional to quote ``comoving separations'' as they
would be now.)
The two competing models for the origin of the
primeval density perturbations involve the physics of the early Universe.
The first holds that around
The competing theory holds that the density perturbations
were seeded by topological defects formed even earlier (
It is the gravitational effects of such defects that would induce
perturbations thousands of years later in the matter distribution.
Although these perturbations would also be approximately scale invariant
the power spectrum of CMB anisotropy would be very different from what
we expect from inflation, because density perturbations would have originated
so much later than in the inflationary scenario.
The current anisotropy data appear to be consistent with inflation and
inconsistent with the topological defect scenario
(see Fig.2).
Both inflation and defect models require nonbaryonic
dark matter.
So do the dynamical measurements
of galaxies and clusters that indicate
there is much more gravitating matter than can be accounted for by
luminous objects or even by dark baryons. The notably successful
theory of big-bang nucleosynthesis
constrains the baryon density to be
less than 100f the ``critical mass density'' above which the Hubble
expansion would eventually become a contraction.
But the dynamical observations indicate that dark matter contributes
at least 200f the critical density, and inflation favors precisely
the critical density.
The observed level of CMB anisotropy provides additional circumstantial
evidence:
If there were only baryons, the level of primeval inhomogeneity required
to produce the observed structure would lead to an anisotropy that is
about ten times larger than that observed.
(See Box 1.)
The non-baryonic matter may be ``cold'' (slow moving) or
``hot'' (fast).
If most of the dark matter is cold, then structure forms
hierarchically - from galaxies to clusters of galaxies to superclusters;
if it is mostly hot, then superclusters form first and then fragment into
clusters and galaxies.
There is now good evidence that galaxies formed first (the bulk around
redshifts of two to three) and that clusters of galaxies and superclusters
formed later, which strongly favors the (mostly) cold dark matter picture.
This, together with measurements of CMB anisotropy, have made inflation+cold
dark matter the working hypothesis for how structure formed in the Universe.
The precise shape of the angular power spectrum depends not only on the
underlying inflation model, but also, in a well understood way, on
cosmological parameters such as the Hubble constant, the mass density and
the composition of the dark matter.
(See Box 2.)
Therefore, the 2500 or so independent multipoles that can be measured have
enormous potential to determine cosmological parameters and to test
theories of the early Universe.
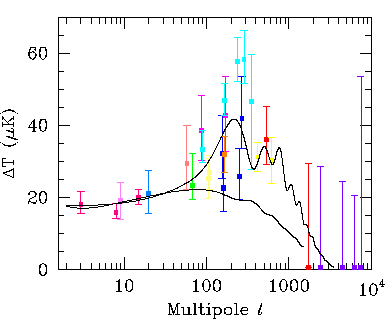 Fig.2:
Angular power spectrum of CMB temperature fluctuations.
The spherical-harmonic multipole number,
Fig.2:
Angular power spectrum of CMB temperature fluctuations.
The spherical-harmonic multipole number, ![]() , is conjugate
to the separation angle
, is conjugate
to the separation angle ![]() .
The data points thus far favor the theoretical expectations for
inflation+cold dark matter (upper curve) over those for
topological defect theories (lower curve, provided by Uros Seljak).
.
The data points thus far favor the theoretical expectations for
inflation+cold dark matter (upper curve) over those for
topological defect theories (lower curve, provided by Uros Seljak).
![]() after the Big-Bang,
a very short burst of tremendous expansion (called inflation) stretched
quantum fluctuations on subatomic scales
to astrophysical size and that these fluctuations
became density perturbations when the vacuum energy that
drove inflation decayed into radiation and matter.
According to this inflationary scenario, the density
perturbations are almost ``scale invariant:'' That is to say,
fluctuations in the
gravitational potential were the same magnitude (
after the Big-Bang,
a very short burst of tremendous expansion (called inflation) stretched
quantum fluctuations on subatomic scales
to astrophysical size and that these fluctuations
became density perturbations when the vacuum energy that
drove inflation decayed into radiation and matter.
According to this inflationary scenario, the density
perturbations are almost ``scale invariant:'' That is to say,
fluctuations in the
gravitational potential were the same magnitude ( ![]() )
on all scales.
Figure 2 shows the angular power spectrum
predicted by inflation.
)
on all scales.
Figure 2 shows the angular power spectrum
predicted by inflation.
![]() )
in a cosmological phase transition associated with spontaneous
symmetry breaking in the theory that unifies the fundamental
forces and particles. Depending upon how the symmetry is broken,
these defects might be
point-like (global monopoles), one-dimensional (cosmic strings), or
three-dimensional (spacetime textures).
)
in a cosmological phase transition associated with spontaneous
symmetry breaking in the theory that unifies the fundamental
forces and particles. Depending upon how the symmetry is broken,
these defects might be
point-like (global monopoles), one-dimensional (cosmic strings), or
three-dimensional (spacetime textures).
![]()
![]()
![]()
Next: Mapping to MicroKelvin Precision
Up: Rosetta Stone
Previous: From Gamow to COBE